Take me to the Steps

A number of interesting assembly puzzles can be made from pieces consisting of simply joined cubes in various numbers and arrangements. Piet Hein’s Soma Cube is a notable example, consisting of all the simply joined non-convex polycubes having four or fewer units. Generally, a polyomino or polycube puzzle is presented as an outline or volume to be filled in with a certain set of pieces. It is up to the solver to figure out how to pack the pieces to fill the specified form.

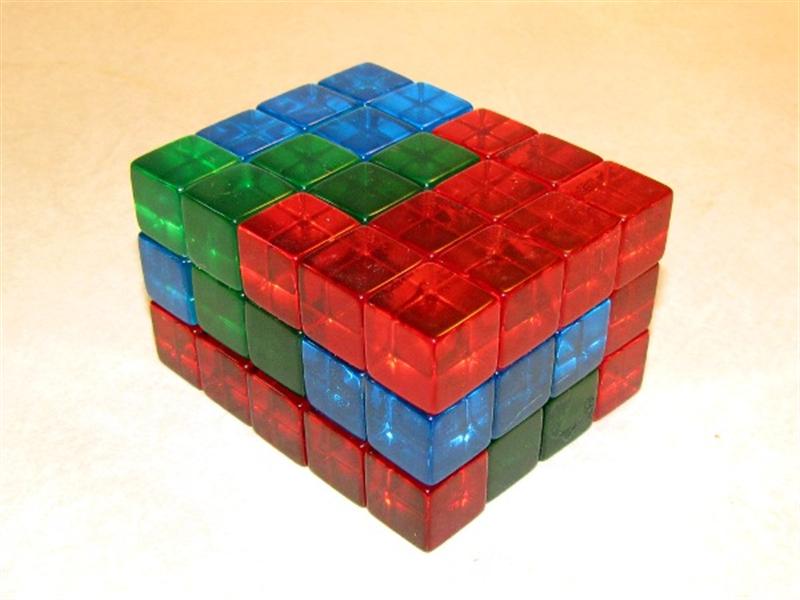
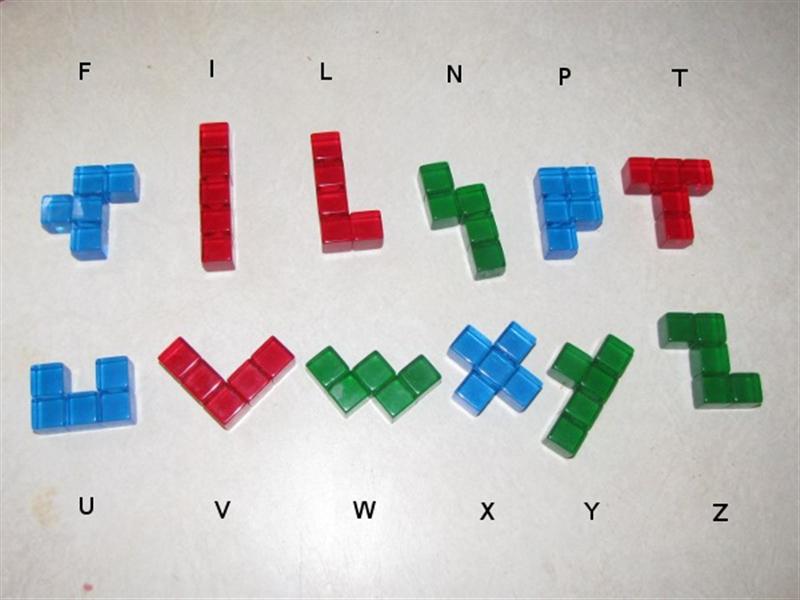
Among the more interesting of the polycube puzzles are the solid pentominoes. The flat pentominoes are commonly used in early elementary education programs, so many readers will doubtless be familiar with them. Extruding the flat pentominoes by one unit in the Z-dimension gives the set of what are traditionally called “solid pentominoes.” They can be used to solve any flat pentomino puzzle, but also to create various 3D shapes. The 3D puzzles are considerably more challenging.

To make a satisfying polycube puzzle requires that the pieces be dimensioned very accurately, so they will always pack closely regardless of their arrangement. To achieve this accuracy with common hand tools is very difficult. However, blank dice provide a convenient and inexpensive source of accurate, precise unit cubes which may be joined to create the various pieces. The use of translucent dice is recommended, both because they look cool and because they’re gauranteed to be acrylic and hence strongly bondable with standard acrylic cements. All the opaque dice I’ve tried to glue have proven highly resistant to adhesives of all types; I suspect they’re made out of polyethylene.

What will the next generation of Make: look like? We’re inviting you to shape the future by investing in Make:. By becoming an investor, you help decide what’s next. The future of Make: is in your hands. Learn More.

Project Steps

Wash dice

Properly cemented acrylic joints are extremely tough. And a large part of getting a good joint is making sure the mating surfaces are free from dirt, oil, and other contaminants that can interfere with the bond. It’s worth it, in the long run, to take the time to wash the blank dice with soap and water.

Finish up with a quick dunk in rubbing alcohol to accelerate drying.

Set up gluing jig

To make true joints, you need a true edge and a true 90-degree angle. This combination square from Harbor Freight (#32244) provides both in an inexpensive tool. The work is done on a steel tray or cookie sheet, so that small supermagnets can be used to hold everything in place while the cement is applied.

Except for the L and V, most pentominoes cannot be glued in a single step against a right angle. Save the L and the V for last, and use their pieces as “blanks” to build up the surface of the angle as needed for each shape.

Glue

To make a piece, the dice are set up against the jig in the proper arrangement and secured in place with magnets. Then a small brush soaked in acrylic cement is applied to each joint, and capillary action draws the cement across the faces to be bonded.

If you are using “blanks,” obviously, be sure to give the joints you do not want glued a wide berth.

Give each piece at least one hour to dry before removing it. Then flip it over and apply cement to each joint, again, from the other side.

Set the pieces aside overnight to cure.

Enjoy!

Pentominoes are a classic mathematical recreation, and there’s lots of information out there about them, including many proven problems. Generally, it’s easiest to start with the “plane” pentomino problems in 2D, a set of which is given above. Again, the idea here is to make the given shape, exactly, using all 12 pentominoes. Another common challenge is to build a 300% scale model of any particular pentomino using 9 of the OTHER pentominoes.

A final challenge is to produce puzzles of your own. There are 12 pentominoes with an area/volume of 5 units each, so any complete pentomino puzzle will have an area/volume of 60 units.

Once you get a feel for the plane problems, you may want to move on to 3D solid pentomino problems, which are considerably more difficult for most people. A set of graded problems is presented below. The difficulty ratings assigned are relative and are based on the number of possible solutions; those rated “hard” have the fewest solutions, and those rated “easy” have the most.

Conclusion

Be careful, when building the U pentomino, to be certain to use a spacer die in the hollow of the U when you glue. Otherwise you may end up with a stubborn U that will accept another block only reluctantly.

A classic book on polyomino puzzles of all types is Solomon W. Golomb's Polyominoes published in 1965. It's interesting reading, if you want an in-depth study of the problem and a gaggle of interesting problems, but it's by no means necessary. There's more than enough polyomino information on the web to satisfy all but the most ardent curiosity.