Early in the education of every mechanical and civil engineer comes a period of long and detailed study of how forces cause things to move or not move. When you’re talking about a car or an airplane, you want those forces to make the thing move, and the general term used to describe this is dynamics. For a bridge, a cellphone tower, or any other thing we don’t want to move, we turn to the study of statics.
Whether moving or standing, a real-world thing can have a bewildering multitude of forces and loads acting on it, such as tension, compression, shear, and torsion. Add in the twisting forces of various torques (or moments of force) on an object, and the design of real-world things becomes a bit difficult. Luckily, the great minds of the past solved some of these difficult problems.
Louis Poinsot was one of those geniuses upon whose work our built-up world is largely made. A French mathematician of the 19th century, he spent his career in the ivory towers of academia formulating the difficult-sounding, yet oh-so-important, field of geometrical mechanics.
Poinsot was the first to demonstrate that any number of individual forces pushing or pulling on a rigid thing can be simplified into just a single linear force and a twisting force called a couple. The great value of the idea, according to Poinsot himself, is that it allowed engineers to think of the motion that a large and complex rigid body undergoes in terms that are much easier to work with.
Why is this important? Consider the problems facing the engineer who attempts to design the hull of a sailing ship or the vanes of a windmill. Winds acting on these objects come from all directions, while frictional forces act on them too, as the vanes and hull slice through air and water. These forces and torques are of myriad magnitudes and directions. Optimizing the design might seem an intractable problem, given the complex interplay of forces.
But thanks to Poinsot, it’s possible to understand what’s going on. Poinsot figured out that all the forces acting on vanes, mast, sails, and keels can be manipulated mathematically, and instead of dealing with a hundred different quantities, you can use vector algebra to reduce them all down to a single resultant linear force and a couple. This simplification was a breakthrough in the field of engineering and made possible the design of all sorts of complicated, moving, spinning things, from submarines and Mars rockets to the liquid-crystal display in your smartphone or flat-screen TV.
The Dancing Spheres
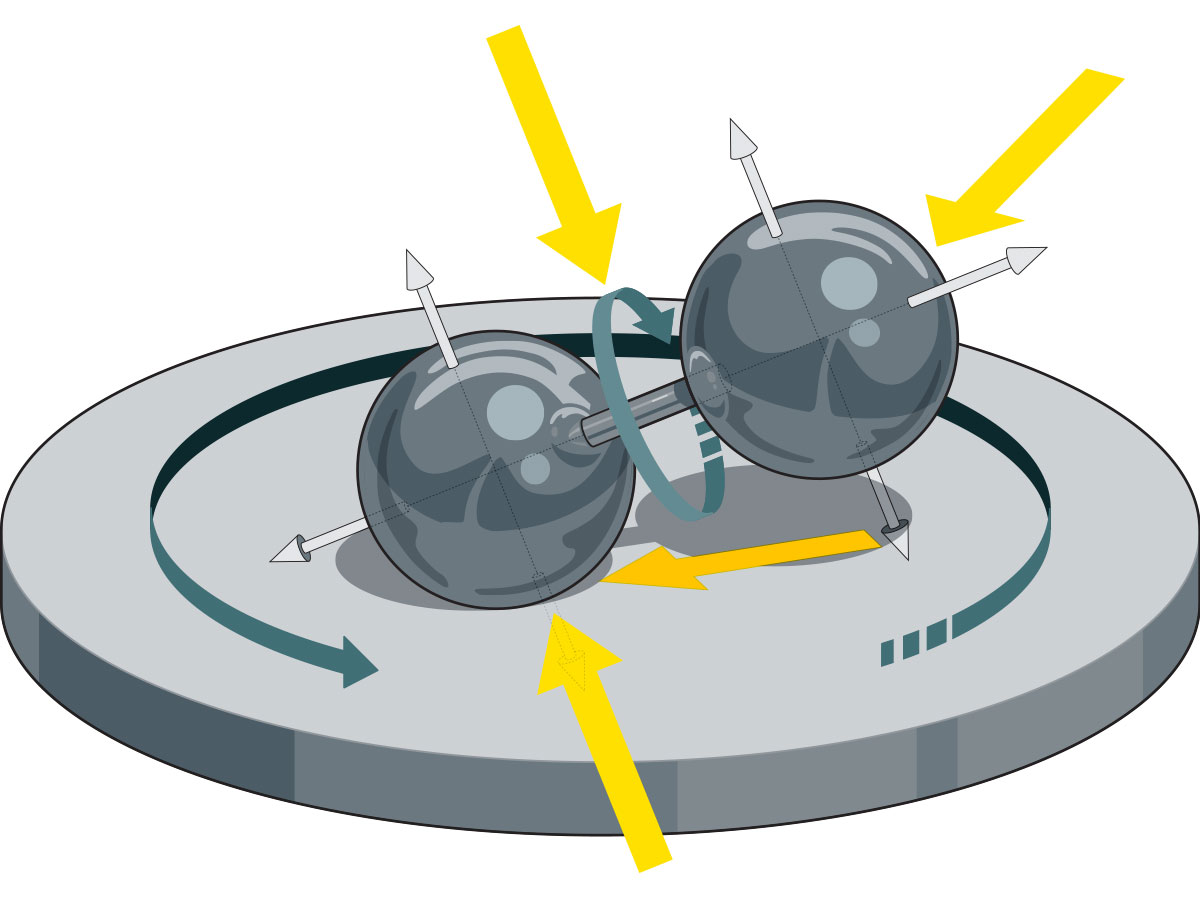
Here’s a fun and novel demonstration of Poinsot’s forces and couples at work. It’s sometimes sold as a science toy called “hurricane balls” or the like. Connect two small steel balls by drilling a short hole in each and connecting them with a short steel or wooden rod. Place them on a flat metal pan and give them a flick with your fingers to make them spin merrily around at amazingly fast rotational speeds.
Now comes the interesting part. If you blow a jet of air on them, the balls’ angular velocity reaches almost unbelievable speeds — thousands of RPMs! You’ve really got to see it; check out the video below.
The system of steel balls interacting with the pan surface, and the force and couple acting on them, is very complex — at high speeds, the force of gravity becomes negligible compared to the toy’s enormous torques — but by using the work of Poinsot, it can be modeled, analyzed, and understood.